Clifford analysis of anisotropic coupled elastostatics and elastodynamics

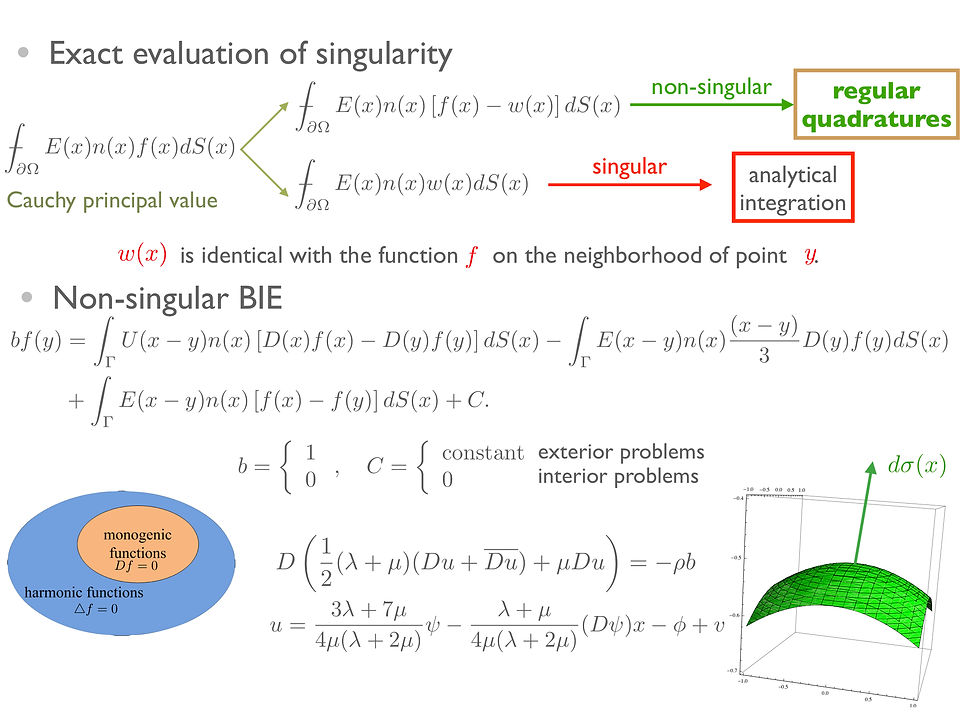
Clifford algebra and Clifford analysis which are the n-dimensional extension of complex algebra and complex analysis are used to study the problems of isotropic and anisotropic elastostatics and elastodynamics in three dimensional space taking into account coupled effect. We will attempt to derive Clifford-valued general solutions from reformulating the governing equations of different problems in terms of the language of Clifford analysis. In order to attack the realistic problems of the elastostatics and elastodynamics with any shape of domains and complicated boundary conditions, we will develop Clifford algebra valued boundary integral equations and Clifford algebra valued boundary element methods by virtue of the closed connection of Clifford analysis and differential form. In both theoretical and computational development of Clifford analysis of anisotropic coupled elastostatics and elastodynamics, we will endeavour to propose the Clifford analysis version of the Cauchy-Riemann operator, the holomorphic function, the Taylor series, the Laurent series, the conformal transformations, and the integral theorems in order to help us to deal with the coupled problems of elastostatics and elastodynamics in three dimensional space.